Page 14 - Read Online

Page 10 of 15 Fan et al. Complex Eng Syst 2023;3:5 I http://dx.doi.org/10.20517/ces.2023.04

$SSUR[LPDWH
+DOLPWRQLDQ

,PSURYHG
XSGDWH UXOH

y y J y
Ö

$XJPHQWHG i i &ULWLF QHWZRUN i i
G\QDPLFV S
Ö

u y
i i &RQWURO
IXQFWLRQ
Ö

v y
i i 'LVWXUEDQFH
IXQFWLRQ
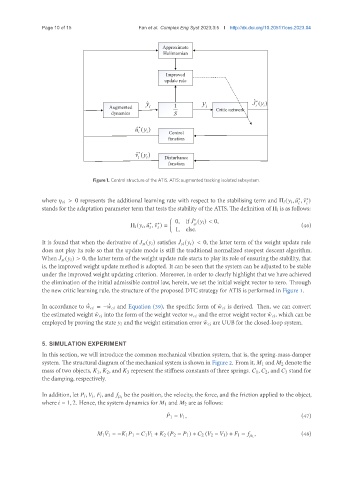
Figure 1. Control structure of the ATIS. ATIS: augmented tracking isolated subsystem.

∗ ∗
where > 0 represents the additional learning rate with respect to the stabilising term and Π ( , ˆ , ˆ )

stands for the adaptation parameter term that tests the stability of the ATIS. The definition of Π is as follows:

¤ ∗
0, if ( ) < 0,
∗
∗
Π ( , ˆ , ˆ ) = 1, else. (46)

It is found that when the derivative of ( ) satisfies ( ) < 0, the latter term of the weight update rule
¤
does not play its role so that the update mode is still the traditional normalized steepest descent algorithm.
When ( ) > 0, the latter term of the weight update rule starts to play its role of ensuring the stability, that
¤
is, the improved weight update method is adopted. It can be seen that the system can be adjusted to be stable
under the improved weight updating criterion. Moreover, in order to clearly highlight that we have achieved
the elimination of the initial admissible control law, herein, we set the initial weight vector to zero. Through
the new critic learning rule, the structure of the proposed DTC strategy for ATIS is performed in Figure 1.
In accordance to ˜ = − ˆ and Equation (39), the specific form of ˜ is derived. Then, we can convert
¤
¤
the estimated weight ˆ into the form of the weight vector and the error weight vector ˜ , which can be
employed by proving the state and the weight estimation error ˜ are UUB for the closed-loop system.

5. SIMULATION EXPERIMENT
In this section, we will introduce the common mechanical vibration system, that is, the spring-mass-damper
system. The structural diagram of the mechanical system is shown in Figure 2. From it, 1 and 2 denote the
mass of two objects, 1, 2, and 3 represent the stiffness constants of three springs. 1, 2, and 3 stand for
the damping, respectively.

be the position, the velocity, the force, and the friction applied to the object,
In addition, let , , , and
where = 1, 2. Hence, the system dynamics for 1 and 2 are as follows:

1 = 1 , (47)
¤

¤ , (48)
1 1 = − 1 1 − 1 1 + 2 ( 2 − 1 ) + 2 ( 2 − 1 ) + 1 − 1