Page 133 - Read Online

Page 4 of 10 Prescott et al. Vessel Plus 2019;3:13 I http://dx.doi.org/10.20517/2574-1209.2018.70

A B

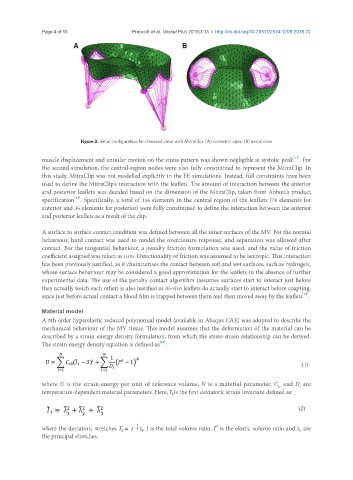
Figure 2. Initial configuration for diseased valve with MitraClip: (A) isometric view; (B) aerial view

[13]
muscle displacement and annular motion on the stress pattern was shown negligible at systolic peak . For
the second simulation, the central-region nodes were also fully constrained to represent the MitraClip. In
this study, MitraClip was not modelled explicitly in the FE simulations. Instead, full constraints have been
used to define the MitraClip’s interaction with the leaflets. The amount of interaction between the anterior
and posterior leaflets was decided based on the dimension of the MitraClip, taken from Abbott’s product
[14]
specification . Specifically, a total of 114 elements in the central region of the leaflets (78 elements for
anterior and 36 elements for posterior) were fully constrained to define the interaction between the anterior
and posterior leaflets as a result of the clip.

A surface to surface contact condition was defined between all the inner surfaces of the MV. For the normal
behaviour, hard contact was used to model the overclosure response, and separation was allowed after
contact. For the tangential behaviour, a penalty friction formulation was used, and the value of friction
coefficient assigned was taken as 0.05. Directionality of friction was assumed to be isotropic. This interaction
has been previously justified, as it characterises the contact between soft and wet surfaces, such as hydrogels,
whose surface behaviour may be considered a good approximation for the leaflets in the absence of further
experimental data. The use of the penalty contact algorithm (assumes surfaces start to interact just before
they actually touch each other) is also justified as in-vivo leaflets do actually start to interact before coapting,
[15]
since just before actual contact a blood film is trapped between them and then moved away by the leaflets .

Material model
A 5th order hyperelastic reduced polynomial model (available in Abaqus CAE) was adopted to describe the
mechanical behaviour of the MV tissue. This model assumes that the deformation of the material can be
described by a strain energy density formulation, from which the stress-strain relationship can be derived.
[16]
The strain energy density equation is defined as :

(1)

where U is the strain energy per unit of reference volume, N is a material parameter, C and D are
i0
i
temperature-dependent material parameters. Here, is the first deviatoric strain invariant defined as:
(2)

where the deviatoric stretches , J is the total volume ratio, J is the elastic volume ratio and l are
el
i
the principal stretches.