Page 22 - Read Online

Schiavone et al. Modelling of metallic and polymeric stents

(insensitive to volumetric deformation). However, Nolan Nevertheless, the HGO model used in this work
et al. found that the HGO-C model was unable to was calibrated properly against the longitudinal and
[26]
[25]
simulate compressible anisotropic behavior correctly, circumferential tensile data of arteries. It was proved
because it used isochoric anisotropic invariants which to be a reliable anisotropic formulation for modeling
is insensitive to volumetric deformation. Consequently, deformation of the arterial layers with collagen fibre
they formulated a modified anisotropic (MA) model by reinforcement.
using the full anisotropic invariants which accounted
for a volumetric anisotropic contribution. The MA Crimping simulation
model correctly predicted the material’s anisotropic To model stent crimping, twelve rigid plates were
response to hydrostatic tensile loading, pure shear generated around the stent as shown in Figure 5. The
and uniaxial deformations. They also found the HGO-C rigid plates were modelled as shell surfaces. Uniform
model significantly underpredicted arterial compliance, radial displacement, linearly increasing to 1 mm within
which might affect the simulation results of stent a step time of 0.1 s, was applied to the plates to enforce
deployment in diseased arteries. To fully clarify this the crimping of stent. Spring back of the stent after
effect, a considerable amount of new work is required, crimping, due to the recovery of elastic deformation,
especially the efforts required for coding a user-defined resulted in a final stent diameter of 1.5 mm, which is
material subroutine for the MA model (interface with the able to fit in the diseased vessel.
FE package Abaqus). This is beyond the scope of this
paper, and will be investigated in our future studies. Abaqus explicit was adopted for crimping simulations
(0.1 s step time). No constraint was applied to the stent.
Hard contact was assigned between the outer surface
of the stent and the rigid plates. The friction coefficient
was assumed to be 0.8. Following crimping, an
additional step was used to simulate the spring back of
the stent within 0.1 s. In this step, the contacts between
the rigid plates and the stent were deleted, allowing for
the stent to recover the elastic deformation freely.

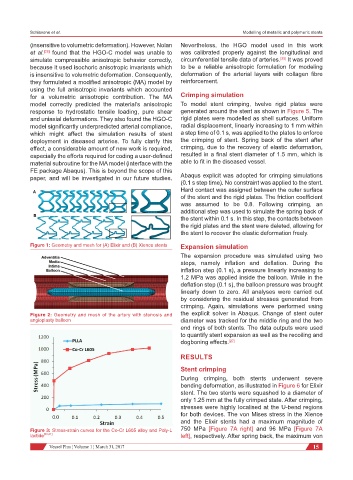
Figure 1: Geometry and mesh for (A) Elixir and (B) Xience stents Expansion simulation
The expansion procedure was simulated using two
steps, namely inflation and deflation. During the
inflation step (0.1 s), a pressure linearly increasing to
1.2 MPa was applied inside the balloon. While in the
deflation step (0.1 s), the balloon pressure was brought
linearly down to zero. All analyses were carried out
by considering the residual stresses generated from
crimping. Again, simulations were performed using
Figure 2: Geometry and mesh of the artery with stenosis and the explicit solver in Abaqus. Change of stent outer
angioplasty balloon diameter was tracked for the middle ring and the two
end rings of both stents. The data outputs were used
to quantify stent expansion as well as the recoiling and
dogboning effects. [27]

RESULTS

Stent crimping
During crimping, both stents underwent severe
bending deformation, as illustrated in Figure 6 for Elixir
stent. The two stents were squashed to a diameter of
only 1.25 mm at the fully crimped state. After crimping,
stresses were highly localised at the U-bend regions
for both devices. The von Mises stress in the Xience
and the Elixir stents had a maximum magnitude of
Figure 3: Stress-strain curves for the Co-Cr L605 alloy and Poly-L 750 MPa [Figure 7A right] and 96 MPa [Figure 7A
lactide [20,21] left], respectively. After spring back, the maximum von
Vessel Plus ¦ Volume 1 ¦ March 31, 2017 15