Page 69 - Read Online

Zhang et al. Intell Robot 2022;2(4):371­90 I http://dx.doi.org/10.20517/ir.2022.26 Page 375

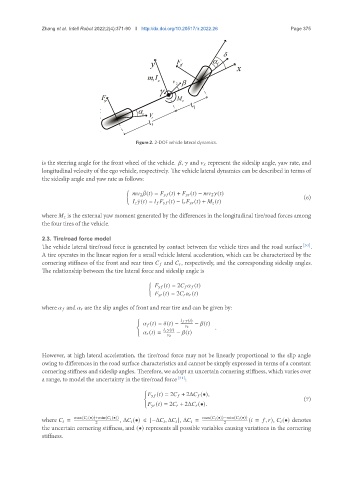
Figure 2. 2-DOF vehicle lateral dynamics.

is the steering angle for the front wheel of the vehicle. , and represent the sideslip angle, yaw rate, and
longitudinal velocity of the ego vehicle, respectively. The vehicle lateral dynamics can be described in terms of
the sideslip angle and yaw rate as follows:

{
¤
2 ( ) = ( ) + ( ) − 2 ( )
(6)
¤ ( ) = ( ) − ( ) + ( )
where is the external yaw moment generated by the differences in the longitudinal tire/road forces among
the four tires of the vehicle.

2.3. Tire/road force model
The vehicle lateral tire/road force is generated by contact between the vehicle tires and the road surface [30] .
A tire operates in the linear region for a small vehicle lateral acceleration, which can be characterized by the
cornering stiffness of the front and rear tires and , respectively, and the corresponding sideslip angles.
The relationship between the tire lateral force and sideslip angle is

{
( ) = 2 ( )
( ) = 2 ( )
where and are the slip angles of front and rear tire and can be given by:

{
( )
( ) = ( ) − − ( )
2 .
( ) = ( ) − ( )
2
However, at high lateral acceleration, the tire/road force may not be linearly proportional to the slip angle
owing to differences in the road surface characteristics and cannot be simply expressed in terms of a constant
cornering stiffness and sideslip angles. Therefore, we adopt an uncertain cornering stiffness, which varies over
a range, to model the uncertainty in the tire/road force [31] :

{
( ) = 2 + 2Δ (•),
(7)
( ) = 2 + 2Δ (•).

where = max( (•))+min( (•)) , Δ (•) ∈ [−Δ , Δ ], Δ = max( (•))−min( (•)) ( = , ), (•) denotes
2
2
the uncertain cornering stiffness, and (•) represents all possible variables causing variations in the cornering
stiffness.