Page 117 - Read Online

Page 301 Peng et al. Intell Robot 2022;2(3):298­312 I http://dx.doi.org/10.20517/ir.2022.27

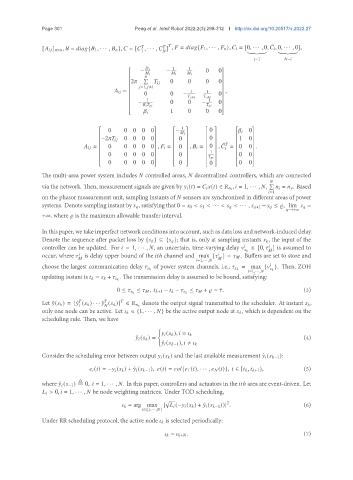
[ ] × , = { 1 , · · · , }, = [ , · · · , ] , = { 1 , · · · , }, = [0, · · · , 0, , 0, · · · , 0],
¯

1
| {z } | {z }
−1 −
1 1
 − − 0 0 
 

 Í 
 2 0 0 0 0 
 =1, ≠ 
 
=  0 0 − 1 1  ,
 0 
ℎ ℎ
 1 1 
 − 0 0 − 0
 
 1 0 0 0 
 
 0 0 0 0 0   − 1   0   0 
       
     0   
 −2 0 0 0 0   0     1 0 
      ¯  
=  0 0 0 0 0 , =  0  , =  0  , =  0 0 .

     1   
 0 0 0 0 0  0     0 0
       
 0 0 0 0 0   0   0   0 0 
       
The multi-area power system includes controlled areas, decentralized controllers, which are connected

Í
, = 1, · · · , , = . Based
via the network. Then, measurement signals are given by ( ) = ( ) ∈ R
=1
on the phasor measurement unit, sampling instants of sensors are synchronized in different areas of power
systems. Denote sampling instant by , satisfying that 0 = 0 < 1 < · · · < < · · · , +1 − ≤ , lim =
→+∞
+∞, where is the maximum allowable transfer interval.
In this paper, we take imperfect network conditions into account, such as data loss and network-induced delay.
Denote the sequence after packet loss by { } ⊆ { }; that is, only at sampling instants , the input of the

controller can be updated. For = 1, · · · , , an uncertain, time-varying delay ∈ [0, ] is assumed to

occur, where is delay upper bound of the ℎ channel and max { } = . Buffers are set to store and

=1,··· ,

= max { }. Then, ZOH
choose the largest communication delay of power system channels, i.e.,
=1,··· ,
. The transmission delay is assumed to be bound, satisfying:
updating instant is = +

≤ + = ¯. (3)
0 ≤ ≤ , +1 − −
denote the output signal transmitted to the scheduler. At instant ,
Let ˆ ( ) = [ ˆ ( ) · · · ˆ ( )] ∈ R
1
only one node can be active. Let ∈ {1, · · · , } be the active output node at , which is dependent on the
scheduling rule. Then, we have
(
( ), =
ˆ ( ) = (4)
ˆ ( −1 ), ≠
Consider the scheduling error between output ( ) and the last available measurement ˆ ( −1 ):
( ) = − ( ) + ˆ ( −1 ), ( ) = { 1 ( ), · · · , ( )}, ∈ [ , +1 ), (5)
where ˆ ( −1 ) ≜ 0, = 1, · · · , . In this paper, controllers and actuators in the ℎ area are event-driven. Let
> 0, = 1, · · · , be node weighting matrices. Under TOD scheduling,
p 2
= arg max | (− ( ) + ˆ ( −1 ))| . (6)
∈{1,··· , }
Under RR scheduling protocol, the active node is selected periodically:

= + . (7)