Page 116 - Read Online

Peng et al. Intell Robot 2022;2(3):298­312 I http://dx.doi.org/10.20517/ir.2022.27 Page 300

b i - 1 D di P Synchronous Power plant
i R governors turbines generator
ACE
+ i + - 1 1 + - 1
Scheduler Network K i i f D
+
+
+ 1 sT+ gi 1 sT ti - D sM i
gain D vi P D P mi D P tiei
N
å T
Tie line ij
j= 1, j i ¹ N
å ij T f D
+ j= 1, j i ¹ j
p
2 /s -
Networked LFC System
Other areas
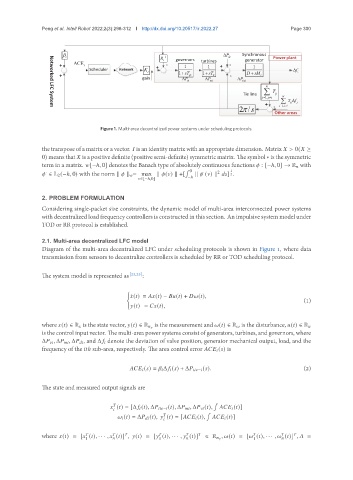
Figure 1. Multi-area decentralized power systems under scheduling protocols.
the transpose of a matrix or a vector. is an identity matrix with an appropriate dimension. Matrix > 0( ≥
0) means that is a positive definite (positive semi-definite) symmetric matrix. The symbol ∗ is the symmetric
term in a matrix. [−ℎ, 0] denotes the Banach type of absolutely continuous functions : [−ℎ, 0] → R with
¯ 0 1
2
·
· k ( ) k ] 2 .
∈ L 2 (−ℎ, 0) with the norm k k = max k ( ) k +[ −ℎ
∈[−ℎ,0]
2. PROBLEM FORMULATION
Considering single-packet size constraints, the dynamic model of multi-area interconnected power systems
with decentralized load frequency controllers is constructed in this section. An impulsive system model under
TOD or RR protocol is established.

2.1. Multi­area decentralized LFC model
Diagram of the multi-area decentralized LFC under scheduling protocols is shown in Figure 1, where data
transmission from sensors to decentralize controllers is scheduled by RR or TOD scheduling protocol.

The system model is represented as [23,25] :

(
¤ ( ) = ( ) − ( ) + ( ),
(1)
( ) = ( ),

where ( ) ∈ R is the state vector, ( ) ∈ R is the measurement and ( ) ∈ R is the disturbance, ( ) ∈ R
is the control input vector. The multi-area power systems consist of generators, turbines, and governors, where
Δ , Δ , Δ , and Δ denote the deviation of valve position, generator mechanical output, load, and the
frequency of the ℎ sub-area, respectively. The area control error ( ) is

( ) = Δ ( ) + Δ − ( ). (2)

The state and measured output signals are

¯

( ) = [Δ ( ), Δ − ( ), Δ , Δ ( ), ( )]

¯

( ) = Δ ( ), ( ) = [ ( ), ( )]

where ( ) = [ ( ), · · · , ( )] , ( ) = [ ( ), · · · , ( )] ∈ R , ( ) = [ ( ), · · · , ( )] , =
1 1 1