Page 8 - Read Online

Page 4 of 11 Liu et al. Microstructures 2023;3:2023009 https://dx.doi.org/10.20517/microstructures.2022.29

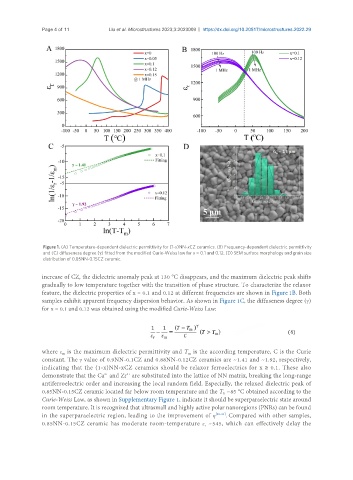
Figure 1. (A) Temperature-dependent dielectric permittivity for (1-x)NN-xCZ ceramics. (B) Frequency-dependent dielectric permittivity
and (C) diffuseness degree (γ) fitted from the modified Curie-Weiss law for x = 0.1 and 0.12. (D) SEM surface morphology and grain size
distribution of 0.85NN-0.15CZ ceramic.

increase of CZ, the dielectric anomaly peak at 130 °C disappears, and the maximum dielectric peak shifts
gradually to low temperature together with the transition of phase structure. To characterize the relaxor
feature, the dielectric properties of x = 0.1 and 0.12 at different frequencies are shown in Figure 1B. Both
samples exhibit apparent frequency dispersion behavior. As shown in Figure 1C, the diffuseness degree (γ)
for x = 0.1 and 0.12 was obtained using the modified Curie-Weiss Law:

where ε is the maximum dielectric permittivity and T is the according temperature, C is the Curie
m
m
constant. The γ value of 0.9NN-0.1CZ and 0.88NN-0.12CZ ceramics are ~1.41 and ~1.92, respectively,
indicating that the (1-x)NN-xCZ ceramics should be relaxor ferroelectrics for x ≥ 0.1. These also
demonstrate that the Ca and Zr are substituted into the lattice of NN matrix, breaking the long-range
4+
2+
antiferroelectric order and increasing the local random field. Especially, the relaxed dielectric peak of
0.85NN-0.15CZ ceramic located far below room temperature and the T ~85 °C obtained according to the
B
Curie-Weiss Law, as shown in Supplementary Figure 1, indicate it should be superparaelectric state around
room temperature. It is recognized that ultrasmall and highly active polar nanoregions (PNRs) can be found
[29-31]
in the superparaelectric region, leading to the improvement of η . Compared with other samples,
0.85NN-0.15CZ ceramic has moderate room-temperature ε ~545, which can effectively delay the
r