Page 65 - Read Online

Li et al. Intell Robot 2021;1(1):58-83 I http://dx.doi.org/10.20517/ir.2021.08 Page 60

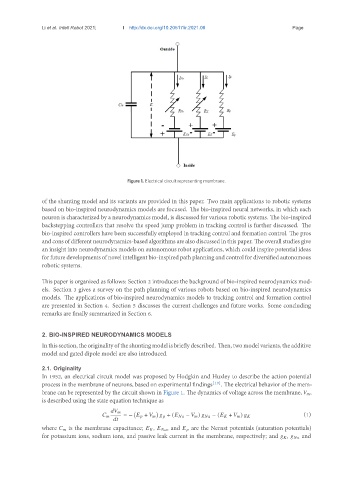
Figure 1. Electrical circuit representing membrane.

of the shunting model and its variants are provided in this paper. Two main applications to robotic systems
based on bio-inspired neurodynamics models are focused. The bio-inspired neural networks, in which each
neuron is characterized by a neurodynamics model, is discussed for various robotic systems. The bio-inspired
backstepping controllers that resolve the speed jump problem in tracking control is further discussed. The
bio-inspired controllers have been successfully employed in tracking control and formation control. The pros
and cons of different neurodynamics-based algorithms are also discussed in this paper. The overall studies give
an insight into neurodynamics models on autonomous robot applications, which could inspire potential ideas
for future developments of novel intelligent bio-inspired path planning and control for diversified autonomous
robotic systems.

This paper is organized as follows: Section 2 introduces the background of bio-inspired neurodynamics mod-
els. Section 3 gives a survey on the path planning of various robots based on bio-inspired neurodynamics
models. The applications of bio-inspired neurodynamics models to tracking control and formation control
are presented in Section 4. Section 5 discusses the current challenges and future works. Some concluding
remarks are finally summarized in Section 6.

2. BIO-INSPIRED NEURODYNAMICS MODELS
Inthissection, theoriginalityoftheshuntingmodelisbrieflydescribed. Then, twomodelvariants, theadditive
model and gated dipole model are also introduced.

2.1. Originality
In 1952, an electrical circuit model was proposed by Hodgkin and Huxley to describe the action potential
process in the membrane of neurons, based on experimental findings [19] . The electrical behavior of the mem-
brane can be represented by the circuit shown in Figure 1. The dynamics of voltage across the membrane, ,
is described using the state equation technique as

( )
= − + + ( − ) − ( + ) (1)

where is the membrane capacitance; , , and are the Nernst potentials (saturation potentials)
for potassium ions, sodium ions, and passive leak current in the membrane, respectively; and , and