Page 79 - Read Online

Li et al. Intell Robot 2021;1(1):58-83 I http://dx.doi.org/10.20517/ir.2021.08 Page 74

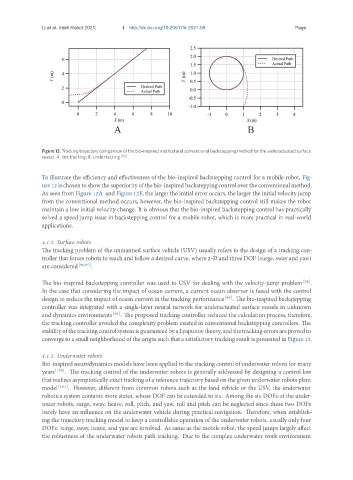
Figure 13. Tracking trajectory comparison of the bio-inspired method and conventional backstepping method for the underactuated surface
vessel. A: line tracking; B: circle tracking [96] .

To illustrate the efficiency and effectiveness of the bio-inspired backstepping control for a mobile robot, Fig-
ure12ischosentoshowthesuperiorityofthebio-inspiredbacksteppingcontrolovertheconventionalmethod.
As seen from Figure 12A and Figure 12F, the larger the initial error occurs, the larger the initial velocity jump
from the conventional method occurs, however, the bio-inspired backstepping control still makes the robot
maintain a low initial velocity change. It is obvious that the bio-inspired backstepping control has practically
solved a speed jump issue in backstepping control for a mobile robot, which is more practical in real-world
applications.

4.1.2. Surface robots
The tracking problem of the unmanned surface vehicle (USV) usually refers to the design of a tracking con-
troller that forces robots to reach and follow a desired curve, where 2-D and three DOF (surge, sway and yaw)
are considered [96,97] .

The bio-inspired backstepping controller was used to USV for dealing with the velocity-jump problem [98] .
In the case that considering the impact of ocean current, a current ocean observer is fused with the control
design to reduce the impact of ocean current in the tracking performance [99] . The bio-inspired backstepping
controller was integrated with a single-layer neural network for underactuated surface vessels in unknown
and dynamics environments [96] . The proposed tracking controller reduced the calculation process, therefore,
the tracking controller avoided the complexity problem existed in conventional backstepping controllers. The
stabilityofthetrackingcontrolsystemisguaranteedbyaLyapunovtheory,andthetrackingerrorsareprovedto
converge to a small neighborhood of the origin such that a satisfactory tracking result is presented in Figure 13.

4.1.3. Underwater robots
Bio-inspired neurodynamics models have been applied to the tracking control of underwater robots for many
years [100] . The tracking control of the underwater robots is generally addressed by designing a control law
that realizes asymptotically exact tracking of a reference trajectory based on the given underwater robots plant
model [101] . However, different from common robots such as the land vehicle or the USV, the underwater
robotics system contains more states, whose DOF can be extended to six. Among the six DOFs of the under-
water robots, surge, sway, heave, roll, pitch, and yaw, roll and pitch can be neglected since these two DOFs
barely have an influence on the underwater vehicle during practical navigation. Therefore, when establish-
ing the trajectory tracking model to keep a controllable operation of the underwater robots, usually only four
DOFs: surge, sway, heave, and yaw are involved. As same as the mobile robot, the speed jumps largely affect
the robustness of the underwater robots path tracking. Due to the complex underwater work environment