Page 25 - Read Online

Page 126 of 139 Clédel et al. J Surveill Secur Saf 2020;1:119­39 I http://dx.doi.org/10.20517/jsss.2020.08

level

Performance

0
Time

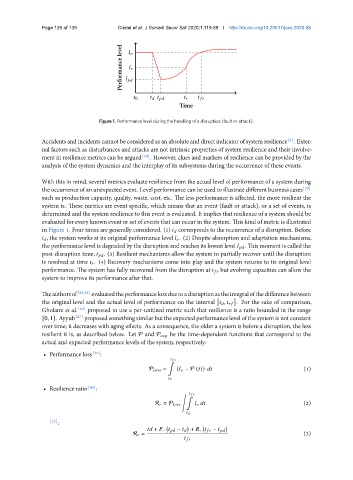
Figure 1. Performance level during the handling of a disruption (fault or attack).

[4]
Accidents and incidents cannot be considered as an absolute and direct indicator of system resilience . Exter-
nal factors such as disturbances and attacks are not intrinsic properties of system resilience and their involve-
ment in resilience metrics can be argued [38] . However, clues and markers of resilience can be provided by the
analysis of the system dynamics and the interplay of its subsystems during the occurrence of these events.

With this in mind, several metrics evaluate resilience from the actual level of performance of a system during
the occurrence of an unexpected event. Level performance can be used to illustrate different business cases [39]
such as production capacity, quality, waste, cost, etc. The less performance is affected, the more resilient the
system is. These metrics are event specific, which means that an event (fault or attack), or a set of events, is
determined and the system resilience to this event is evaluated. It implies that resilience of a system should be
evaluated for every known event or set of events that can occur in the system. This kind of metric is illustrated
in Figure 1. Four times are generally considered. (1) corresponds to the occurrence of a disruption. Before
, the system works at its original performance level . (2) Despite absorption and adaptation mechanisms,
the performance level is degraded by the disruption and reaches its lowest level . This moment is called the
post-disruption time, . (3) Resilient mechanisms allow the system to partially recover until the disruption
is resolved at time . (4) Recovery mechanisms come into play and the system returns to its original level
performance. The system has fully recovered from the disruption at but evolving capacities can allow the
system to improve its performance after that.

Theauthorsof [26,34] evaluatedtheperformancelossduetoadisruptionastheintegralofthedifferencebetween
[ ]
the original level and the actual level of performance on the interval , . For the sake of comparison,
Gholami et al. [40] proposed to use a per-unitized metric such that resilience is a ratio bounded in the range
[0, 1]. Ayyub [27] proposed something similar but the expected performance level of the system is not constant
over time; it decreases with aging effects. As a consequence, the older a system is before a disruption, the less
resilient it is, as described below. Let P and P be the time-dependent functions that correspond to the
actual and expected performance levels of the system, respectively:

• Performance loss [34] :

∫
P = ( − P ( )) (1)

• Resilience ratio [40] :
/
∫
R = P (2)

[27] :
( ) ( )
+ . − + . −
R = (3)